|
Bulb Cant and Other
Comparisons
by Lester Gilbert
In my previous article [Trim
& Drag (AMYA MY #174)], I showed some results from towing
tank experiments in boat trim that Graham Bantock and I ran in the University of
Southampton’s Lamont towing tank. For this article, our experiment investigated
canting an IOM bulb to see how much difference this makes to hull drag. On my
website (Bulb cant), I have reported some
work that Graham and I did in 2003 on the theory behind bulb cant (Bantock &
Gilbert, 2003). We concluded that a typical IOM bulb should ideally be placed
nose-up by around 2 to 2.5 degrees. We wondered how much extra drag a bulb might
cause if it was excessively canted, so we compared a
normal bulb cant of about 2 degrees
with a cant of about 5 degrees on a Fraktal design IOM. We also wondered how
much drag a normally canted bulb, say, canted at 2 to 2.5 degrees, caused in the
first place. So we ran a series of tests with the bulb canted
normally, with it canted
excessively, and with it not canted
at all. These runs were made with Graham’s falling weight device (FWD), using a
modest drive weight. A boat speed of around 0.48 m/s is about 1 mph, which
corresponds to a light to medium-light
air day at the pond. We did find something interesting.
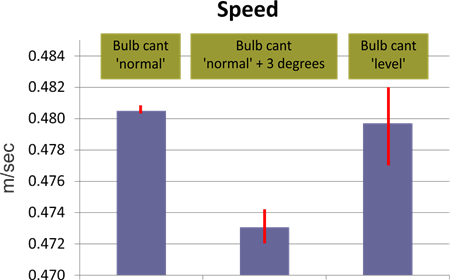
Figure 1. Speed with 0, 2,
and 5 degrees bulb cant
As shown in Figure 1, for a
normal bulb cant of around 2 degrees,
we measured a baseline boat speed of around 0.480 m/s as the average over 3
runs, with a measurement error (standard error of the mean) below 0.001 m/s.
Then, for an excessively canted bulb, the results were as expected, in that the
hull with the bulb canted around 5 degrees showed higher drag and hence lower
speed for a given drive force. With the
excessive bulb cant, speed dropped to an average of 0.473 m/s over 5 runs
(error ±0.0015). What was unexpected was that the boat speed with an uncanted
bulb (0 degrees) was pretty much the same as that with a normally canted bulb (2
degrees). The hull with an uncanted bulb recorded an average speed of 0.480 m/s
as well (over 5 runs, error somewhat higher, but still low, at ±0.003). It seems
that, under the test conditions we used, the bulb cant of 2 degrees did not
trigger stall or laminar flow separation, and in that case its drag would be
difficult to distinguish from an equally unstalled bulb canted at zero degrees.
On the other hand, it is clear that the bulb canted at 5 degrees did trigger
turbulence or laminar flow separation, and hence showed detectably more drag.
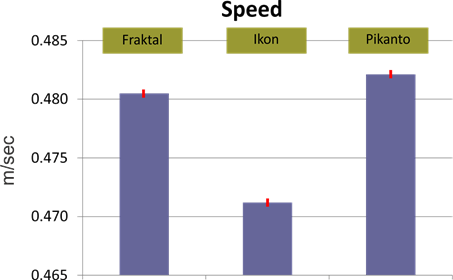
Figure 2. Fraktal, Ikon and
Pikanto speed comparison
The earlier results on hull drag in design-LWL (length of
waterline) trim, using an Ikon, can be nicely compared with the data here for a
Fraktal and a Pikanto. The Ikon and Fraktal designs represent two highly
contrasting approaches to the design of an IOM. The Ikon is a relatively beamy
hull and was designed to contend with the all-conquering TS2 in the late 1990s
and early 2000s. As such, it gives away wetted surface-area drag for the benefit
of increased righting moment in a blow. Since the early 2000s, the trend in IOM
design has been towards narrower hull forms, where speed is not given away in
lighter air, and different sailing techniques are used to compensate for reduced
righting moment in a blow. The Fraktal is an example of a contemporary (2012),
narrow-beam IOM design. Below the (unheeled) waterline, there is not much
difference between the Fraktal and the Pikanto.
Figure 2 shows the Fraktal average speed is around 0.480 m/s,
as we saw above, while the Ikon average speed was 0.471 m/s, as we saw in the
earlier article. The measurement error is quite low, around ±0.001, so we can be
quite confident that the drag of the Fraktal hull under towing tank conditions
is significantly lower than the drag of an Ikon hull. The difference is around
one percent, and though that doesn’t sound like much, it is probably about one
boat length when you round the windward mark. The Pikanto average speed was
0.481 m/s, and we judge that this is not actually higher than the Fraktal speed
of 0.480 m/s, since the difference is within the bounds of measurement error.
Acknowledgements
These experiments would not have been possible without Graham Bantock’s
enthusiasm and knowledge, or without the support of Prof. Philip Wilson of the
University of Southampton’s Department of Ship Science.
Reference
Bantock, Graham and Gilbert, Lester (2003). Some notes on bulb inclination and
on using a general VPP (Velocity Prediction Program) for modeling its effects.
In The Modern Yacht, Southampton, Sep
17–18, 2003, The Royal Institution of Naval Architects, pgs 59–66.
(Added note since original publication: The
towing tests were carried out with the hull upright, floating on its design LWL,
and towed at around 0.5 m/s from a stub mast resulting in negligible pitch.)
|
